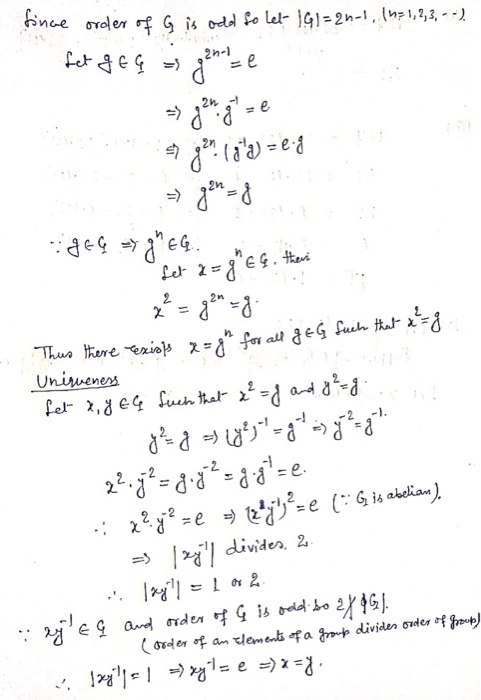Abstract Algebra Groups Problems And Solutions

11 divisors before working through the solved problems for this section you need to make sure that you are familiar with all of the de nitions and theorems in the section. For in depth discussion of abstract algebra go to in depth wikibook on abstract algebra. This page helps students who have encoutered terms from abstract algebra and need to quickly brush up their knowledge.
abstract algebra groups problems and solutions

Problems and solution only the section on groups.

Abstract algebra groups problems and solutions. 34 7 group theory solutions 35 8 galois theory solutions 51 bibliography 61 index 62 iii. This theme covers the basics of working with sets and relations and applying knowledge of logic and proofs. Groups rings fields problem solving forum post your problems or solutions. Online textbooks learn abstract algebra.

Hence eis a left identity. A fundamental and ubiquitous object in all abstract algebra. Aprove that is an equivalence relation. Group composition is a binary operation.

The fourth chapter is the beginning of algebra ii more particularilyit is all about the problems and solutions on field extensionsthe last chapter consists of the problems and solutions in field extensions and galois theoryin most of african universities curriclulumthe last two chapters are given at graduate level. Abstract algebra manual. Iv jabeachy preface preface my goal is to provide some help in reviewing chapters 7 and 8 of our book abstract algebra. Abstract algebra assumes a working prerequisite knowledge of necessary mathematical fundamentals.

Proceedings of a conference held at oxford under the auspices of the science research council atlas computer laboratory 29th august to 2nd september 1967 edited by john leech reader in computing science stirling university with a foreword by dr. Problems in abstract algebra. Blet rz denote the set of equivalence classes of. I have included summaries of most of these sections together with some general comments.

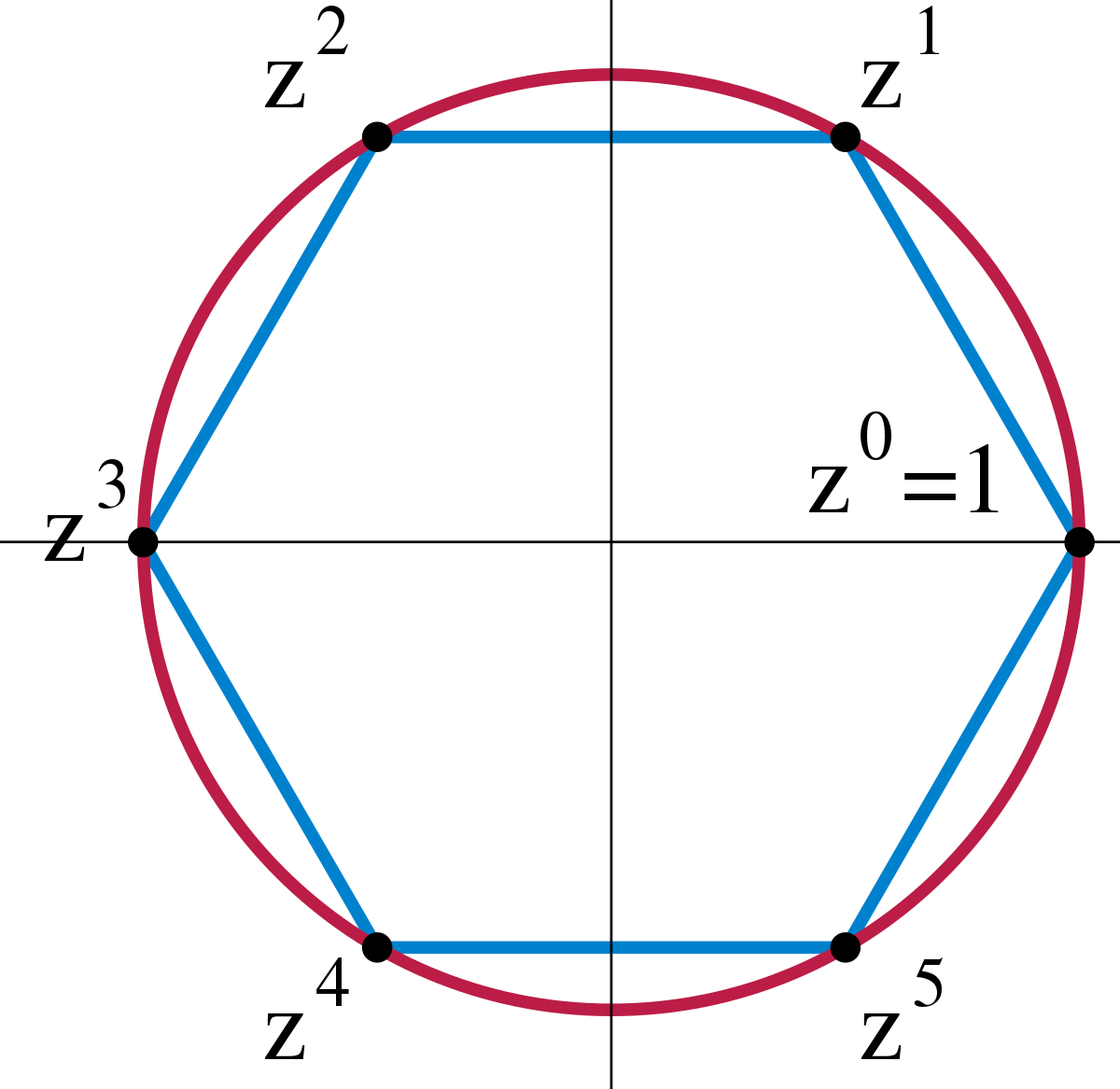
Groups rings fields atexamples. Atlas computer laboratory pergamon press. Cis rz a group. Exercises and solutions in groups rings and fields 5 that yaayat ethen yaae e hence yaa eso every right inverse is also a left inverse.

The review problems are. Everything you will need to know about cyclic groups. Binary operations and closure. In fact although chapter 1 is very concrete it is a signi cant step forward into the realm of abstract algebra.

Prove that the binary operation on rz given by a b a b is well de ned. Now for any a2gwe have ea ayaa ayaa ae aas eis a right identity. Math 1530 abstract algebra selected solutions to problems problem set 2 2de ne a relation on r given by abif a b2z.