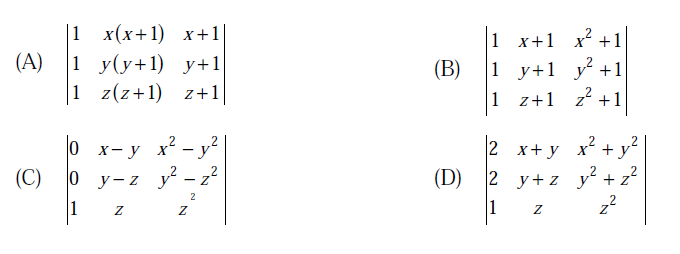Abstract Algebra Associativity Problems And Solutions

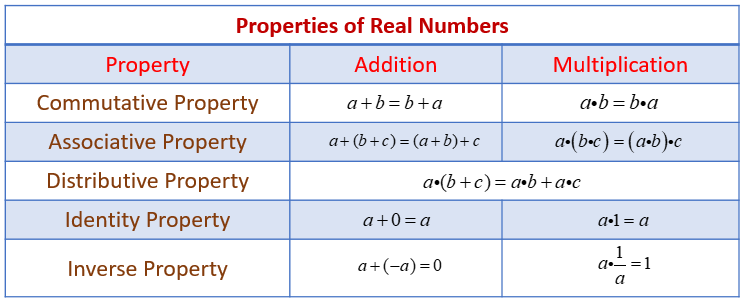
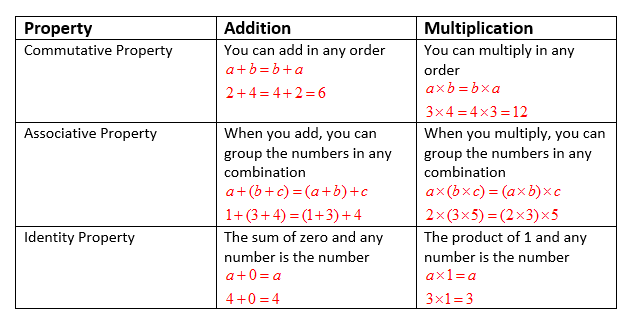
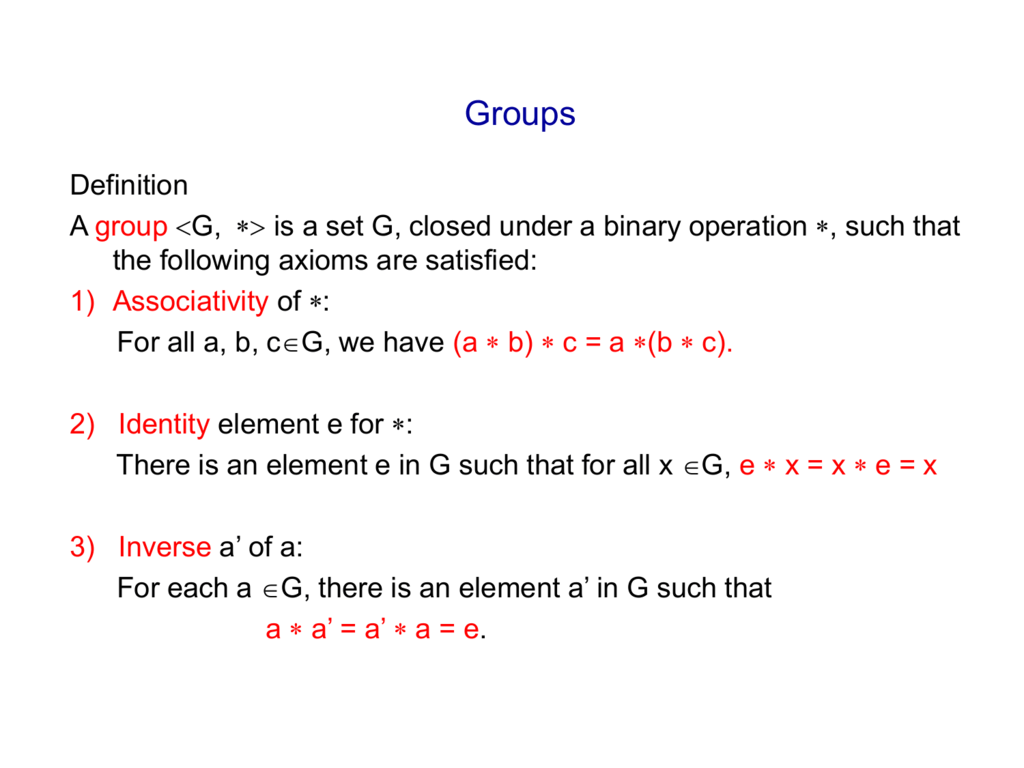
For all ab g the element a b is a uniquely defined element of g.

Abstract algebra associativity problems and solutions. Groups rings fields problem solving forum post your problems or solutions. Applications the question of including applications of abstract algebra in an undergraduate course especially a one semester course is a touchy one. Cis rz a group. Prove that the binary operation on rz given by a b a b is well de ned.

For example the integers endowed with the addition operation form a group. Dene an operation on g by xy xy. The fourth chapter is the beginning of algebra ii more particularilyit is all about the problems and solutions on field extensionsthe last chapter consists of the problems and solutions in field extensions and galois theoryin most of african universities curriclulumthe last two chapters are given at graduate. Let g 01 be the set of real numbers x with 0 x 1.

This page helps students who have encoutered terms from abstract algebra and need to quickly brush up their knowledge. Until recently most abstract algebra texts included few if any applications. Aprove that is an equivalence relation. Either one runs the risk of making a visibly weak case for the applicability of the notions of abstract algebra or on the other handby including substantive.

Instead of just expanding the material that is already written down in our textbook i decided to try to teach by example by writing out solutions to problems. Blet rz denote the set of equivalence classes of. There exists an identity element e g such that. Math 1530 abstract algebra selected solutions to problems problem set 2 2de ne a relation on r given by abif a b2z.

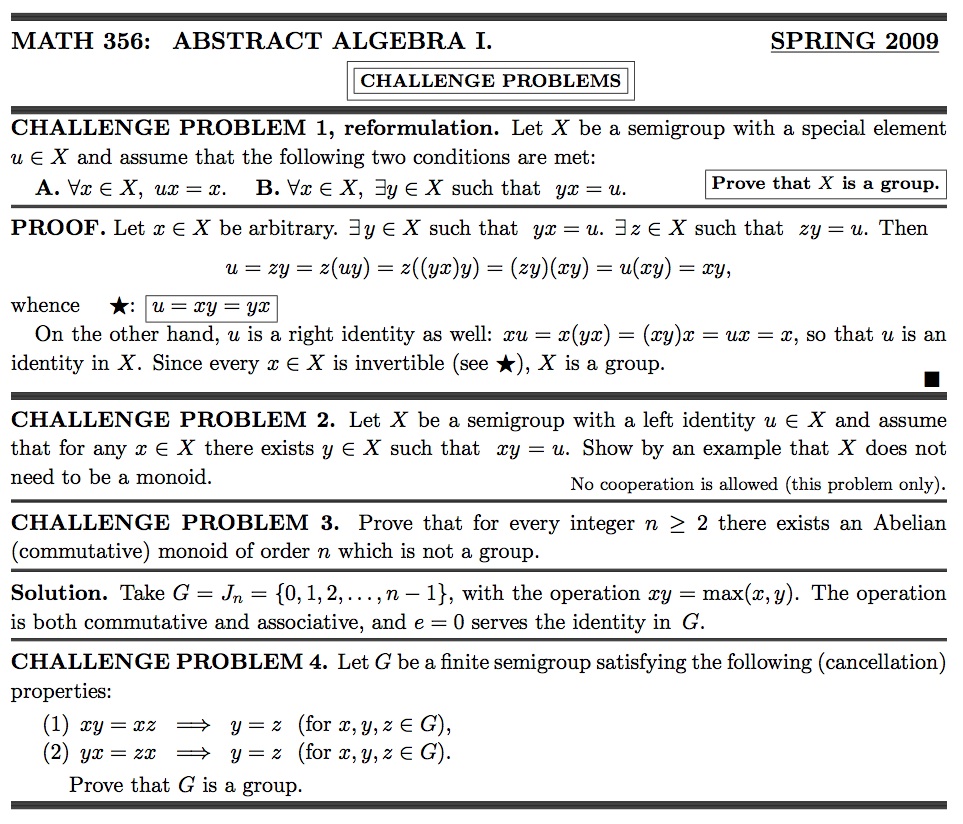
Ive tried to choose problems that would be instructive and in quite a few cases ive included comments to help the reader see what is really going on. Algebra and are discussed next. A group g is a nonempty set g together with a binary operation on g such that the following conditions hold. The group axioms namely closure associativity identity and invertibility.

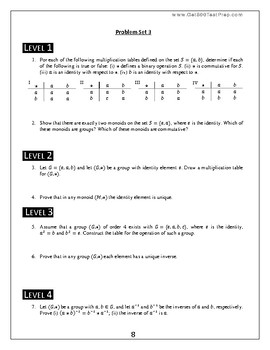
Abstract algebra manual. For all abc g we have a b c a b c. Problems and solution only the section on groups. There are one hundred twenty generators of z 225.

A positive integer a 225 is a generator of z 225 just in case it is divisible by neither 3 nor 5. Online textbooks learn abstract algebra. Subject of abstract algebra and no student should go through such a course without a good notion of what a proof is the importance of applications such as coding theory and cryptography has grown signicantly. Many familiar mathematical structures such as number systems obey these axioms.

Exciting problems on each topic. For in depth discussion of abstract algebra go to in depth wikibook on abstract algebra. However the abstract formalization of the group axioms detached as it is from.